高校数学Ⅱの【三角関数】で学ぶ『2倍角の公式を用いる方程式』について解説!
2倍角の公式を用いて式変形をして,三角関数に関する方程式を解く手順をまとめました!
この投稿を見れば,2倍角の公式を用いる三角関数の方程式はバッチリ!
2倍角の公式

方程式で使う「2倍角の公式」はこの3つ!
$\sin2\alpha=2\sin\alpha\cos\alpha$
$\cos2\alpha=1-2\sin^2\alpha$
$\cos2\alpha=2\cos^2\alpha-1$
「2倍角の公式」詳しくはこれ↓

正弦の2倍角の公式を用いる方程式

$\sin2\alpha=2\sin\alpha\cos\alpha$
を使って問題を解いてみよう!
$\sin2\theta+\cos\theta=0$
$2\sin\theta\cos\theta+\cos\theta=0$
$\cos\theta(2\sin\theta+1)=0$
$\cos\theta=0$ または $\displaystyle{\sin\theta=-\frac{1}{2}}$
$0≦\theta<2\pi$ より
$\cos\theta=0$ を解くと $\displaystyle{\theta=\frac{\pi}{2},\frac{3}{2}\pi}$
$\displaystyle{\sin\theta=-\frac{1}{2}}$ を解くと $\displaystyle{\theta=\frac{7}{6}\pi,\frac{11}{6}\pi}$
以上より
$\displaystyle{\theta=\frac{\pi}{2}, \frac{3}{2}\pi,\frac{7}{6}\pi,\frac{11}{6}\pi}$
$\cos\theta=0$ または $\displaystyle{\sin\theta=-\frac{1}{2}}$ の解き方が分からない場合はこれ↓

余弦の2倍角の公式を用いる問題

$\cos2\alpha=1-2\sin^2\alpha$
$\cos2\alpha=2\cos^2\alpha-1$
を使い分けて問題を解いてみよう!
$\cos2\alpha=1-2\sin^2\alpha$ を用いる問題

$\cos2\alpha$ を $\sin\alpha$ に置き換えたいときは
$\cos2\alpha=1-2\sin^2\alpha$ を使おう!
$\cos2\theta-\sin\theta=0$
$(1-2\sin^2\theta)-\sin\theta=0$
$2\sin^2\theta+\sin\theta-1=0$
$(\sin\theta+1)(2\sin\theta-1)=0$
$\displaystyle{\sin\theta=-1,\frac{1}{2}}$
$0≦\theta<2\pi$ より
$\sin\theta=-1$ を解くと $\displaystyle{\theta=\frac{3}{2}\pi}$
$\displaystyle{\sin\theta=\frac{1}{2}}$ を解くと $\displaystyle{\theta=\frac{\pi}{6},\frac{5}{6}\pi}$
以上より
$\displaystyle{\theta=\frac{\pi}{6}\pi,\frac{5}{6}\pi,\frac{3}{2}\pi}$
$\cos2\alpha=2\cos^2\alpha-1$ を用いる問題

$\cos2\alpha$ を $\cos\alpha$ に置き換えたいときは
$\cos2\alpha=2\cos^2\alpha-1$ を使おう!
$\cos2\theta-3\cos\theta+2=0$
$(2\cos^2\theta-1)-3\cos\theta+2=0$
$2\cos^2\theta-3\cos\theta+1=0$
$(\cos\theta-1)(2\cos\theta-1)=0$
$\displaystyle{\cos\theta=1,\frac{1}{2}}$
$0≦\theta<2\pi$ より
$\cos\theta=1$ を解くと $\theta=0$
$\displaystyle{\cos\theta=\frac{1}{2}}$ を解くと $\displaystyle{\theta=\frac{\pi}{3},\frac{5}{3}\pi}$
以上より
$\displaystyle{\theta=0,\frac{\pi}{3},\frac{5}{3}\pi}$
因数分解に困ったら
「$\sin\theta$ や $\cos\theta$ を含む式の因数分解は計算が難しい」
という人は,置き換えを利用すると計算しやすい。
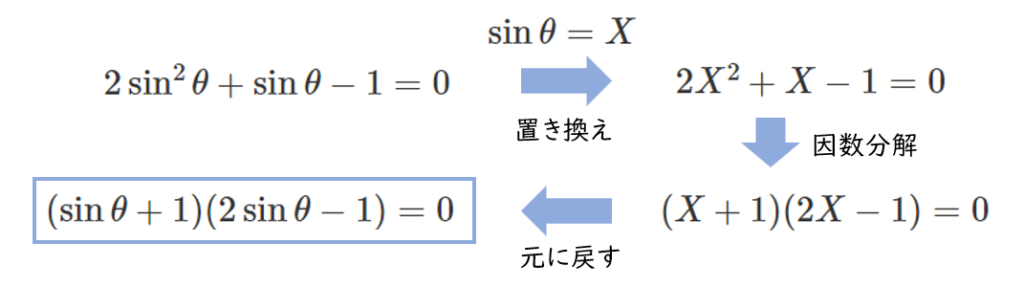
このように,置き換えてから因数分解して元に戻すと計算しやすい。
慣れてきたら,置き換えずにできるようになります。
まとめ
● 三角関数を含む方程式で用いる2倍角の公式
$\sin2\alpha=2\sin\alpha\cos\alpha$
$\cos2\alpha=1-2\sin^2\alpha$
$\cos2\alpha=2\cos^2\alpha-1$
● $\cos2\alpha$ の使い分け
$\sin\alpha$ に書き換えたいときは $\cos2\alpha=1-2\sin^2\alpha$
$\cos\alpha$ に書き換えたいときは $\cos2\alpha=2\cos^2\alpha-1$

使う場面を理解して,「2倍角の公式」を使いこなせるようになろう!




コメント